

And if I move this over 2, then I have to subtract 2 from the exponent, and 10 to the minus 9 is a nanometer. And if we look at the answers all the answers say nanometers, so we need to convert this to nanometers. 8.26 times 10 to the negative 7 and the units are, of course, meters. Alright, punch in all these numbers in your calculator and tell me what you get. And then we have m for this case, because in the problem they say it's the first dark fringe. So we can take the sine of 4.13- or, 4.31 degrees.
So if we have that equation then we might have everything we need, right? Because Jessica said 'well, let's just solve this for lambda, that's what we're looking for.' Lambda equals d sine theta divided by m.
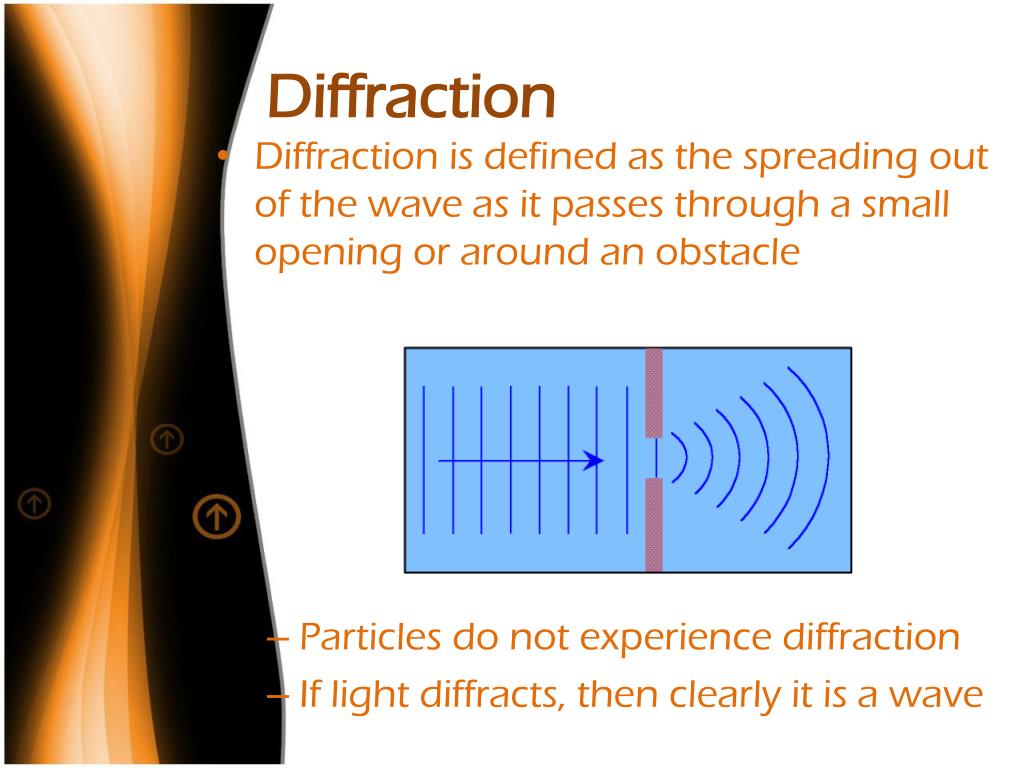
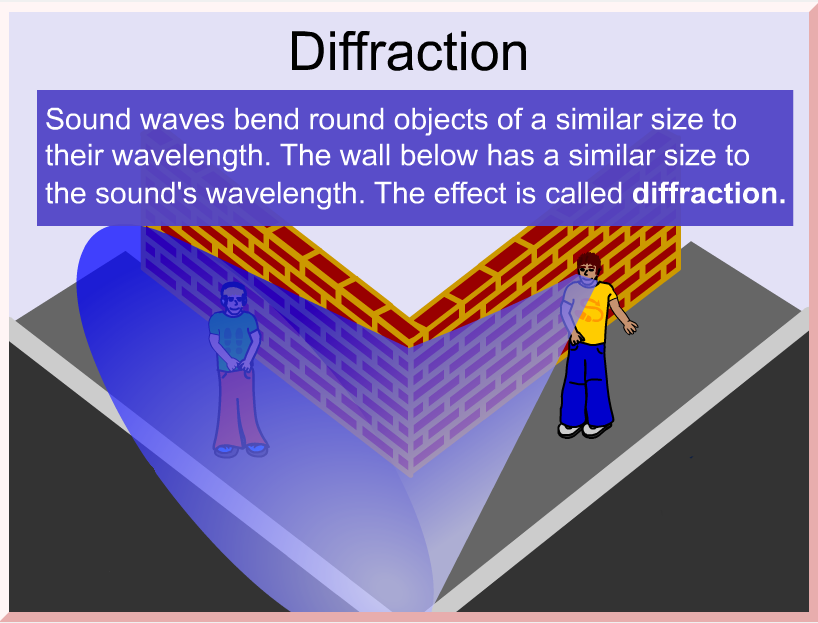
So this is kind of weird, right? But this is the bright fringe for a double slit, it's also the dark fringe for a single slit. Okay, in that equation a is the width and so that's d, and p is an integer, that's our m. That is, in fact, the same equation as this. We get to single slit diffraction, which says the dark fringe is a sine theta equals p lambda. And if you scroll down a little bit more, hopefully, won't let me scroll down. Okay so, you scroll down a little bit on the formula sheet, and we see the bright fringes from Young's double slit certainly obeys that equation d sine theta equals m lambda. So let's pull up the formula sheet off of Blackboard and take a look at what it says. You're going to go to the formula sheet that I posted online for you guys, and you're going to look at that formula sheet and see if that equation is right or not. What are you going to do? You're going to use the same equation and just hope? No you're not. So, is this equation the same or is it something else? Anybody have a thought? How do you deal with this? Let's say you're in the exam tomorrow. Right, and now we have the dark fringe from a single slit. Okay, but this equation that you just told me, that was for the bright fringe from a double slit. Jessica, what should we do? Okay, d sine theta equals m times lambda. So, how do we do this? Somebody give me some thoughts. So here's the center line, and they tell us this angle right here. And they tell us the angle to the first dark fringe. And then they tell us that d is 11 microns, which is 11 times 10 to the minus 6 meters. I think we were calling it- what were we calling it, d? I think I called it w in in lecture but that's fine, we can use d. And the idea is this, we have a single slit, some plane wave coming in, creates this diffraction pattern, and we know that- we know the slit width. So, number six says a beam of monochromatic light passes through a slit that is 11 microns wide, if the first order dark fringe of the resulting diffraction pattern is at an angle of 4.31 degrees away from the center line, what is the wavelength of light? Okay, so this is back to a single slit problem.


 0 kommentar(er)
0 kommentar(er)
